Nicolas Escoubet will defend his PhD on Thursday, March 09th, 2023 at 14h00 in the Georges Charpak amphitheater (Tower 22, Ground floor, Jussieu Campus).

He will present his work on the behavior of the mechanosensitive microswimmer Paramecium in crowded environments, in front of the jury composed of:

- Alexis Prevost, PhD advisor,

- Lea-Laetitia Pontani, co-advisor,

- Romain Brette, co-PhD advisor,

- Xavier Noblin, referee,

- Jean-Paul Rieu, referee,

- Salima Rafai, examinator

- Hélène de Maleprade, examinator,

- Eric Clement, examinator.

Abstract

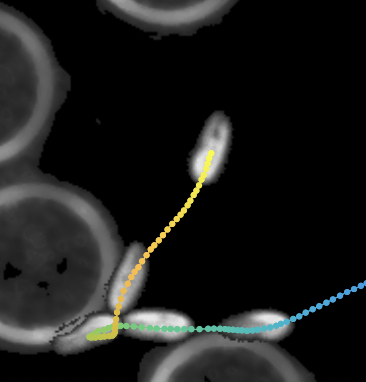
Paramecium is a unicellular eukaryotic ciliate microorganism, about 100 micrometers long, that lives naturally in puddles or ponds and moves by beating the thousands of cilia that cover its surface. Despite its relative simplicity, it can respond to different types of stimuli (chemical, temperature, etc.) thanks to a reorientation mechanism called the Avoiding Reaction (AR). This Avoiding Reaction is also triggered during a mechanical stimulation of its anterior part and results from internal bioelectrical and biochemical processes. In this thesis, we focus our attention on this mechanosensitive behavior. For this purpose, we imaged paramecia swimming freely in pseudo-2D environments with or without obstacles in order to characterize their mechanosensitive properties and to understand how mechanosensitivity affects their swimming behavior. 

In this thesis, we first focused on the behavior of paramecia evolving in an environment without any obstacle. We characterized the local dynamics of an AR, consisting of a backward swimming followed by a reorientation, as well as the statistics of AR on a larger scale and finally how spatial exploration in the absence of any obstacle is affected by AR. In particular, we showed that these spontaneous AR lead to a random walk-like swimming pattern with exponentially distributed step lengths. 

In a second part, we studied the swimming of paramecia in environments with cylindrical obstacles. We observed that upon collision with an obstacle, paramecia were either passively deflected or triggered an AR. This obstacle-triggered AR, a signature of mechanosensitivity, is either triggered instantaneously or delayed. We then quantified the probability of triggering such AR as a function of the swimming speed and the obstacle size. Finally, we highlighted memory effects related to the history of previous collisions. 

In a third part, we used numerical simulations of Active Brownian Particles to study their long time and long distance behavior. We characterized the efficiency of their spatial exploration by diffusion when different parameters were changed, such as the type of AR triggered (no obstacle-triggered AR, no AR at all, any type of AR) or the probability of an obstacle-triggered AR. These simulations suggest that in a periodic network of obstacles, the experimentally measured probability of obstacle-triggered AR coincides with an optimum of diffusion.